(Cross-posted from WBGO.org. Thanks to Nate Chinen for inviting me to write this.)
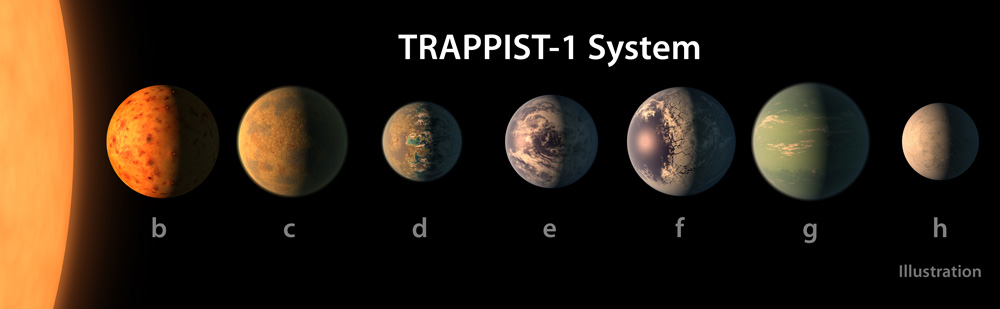
With most things, I’ve found, what’s most interesting isn’t the thing in itself, but rather how it relates to other things. In other words, everything is relative, which is why I was so excited to see the video below. It shows, using harmony, rhythm and visuals, the relationships between the orbits of the seven planets around TRAPPIST-1, a dwarf star about 40 light years away from us.
The video was made by astrophysicist Daniel Tamayo, who worked out the planets’ orbits, and Matt Russo, a fellow scientist who also plays jazz guitar. Musician Andrew Santaguida contributed as well.
What’s going on here? Why does it sound so good? How, in the first place, were these orbits figured out? It’s only in the past 25 years that we’ve been able to detect planets in orbit around stars other than our own. This is mainly done indirectly, by — for example — measuring faint dips in a star’s brightness as planets pass in front of it. In 2015, using this technique, three exoplanets, as planets outside of our Solar System are called, were discovered orbiting around TRAPPIST-1, a dwarf star about 40 light-years away from us in the Aquarius constellation. In February 2017, four more were discovered. The field of exoplanet detection has gotten so advanced that scientists are able to determine not only the size and mass of these planets, but also their orbital periods and — most amazingly — chemical composition. Three of TRAPPIST-1’s planets are considered to be within its habitable zone, where life could potentially be sustained, and some of the planets may even have liquid water on their surface.
This is remarkable enough already. But what makes the TRAPPIST-1 system even more special is the relationships between its planets’ orbits. The farthest planet completes one orbit every 18 Earth days, and the next farthest once every 12. What’s 18 divided by 12? That’s 1.5, or 3/2. In other words, in the time it takes the farthest planet to go twice around the star, the next farthest has gone around three times.
We hear sound when the air around us vibrates and those vibrations reach our ears. Vibrations are a bit like orbits — they repeat at a certain frequency (the note A in the middle of a piano, for example, vibrates 440 times per second). What does it sound like when sounds vibrate in that same 3/2 ratio, one sound vibrating twice in the time it takes the other to vibrate three times? As Pythagoras discovered long ago, it sounds lovely. The musical interval which results, the so-called perfect fifth, is one of the fundamental building blocks of harmony — and not only Western harmony. It turns up in traditional music from all around the world, in every possible key. It sounds like this:
Now let’s look at the closest planet to TRAPPIST-1. It orbits the star in only 1.5 Earth days (these orbital periods are short because the system is very compact — all seven planets orbit the star much closer than Mercury orbits the Sun, and the star itself is only about the size of Jupiter). The next closest goes around once every 2.4 days, which gives their orbits a relationship of about 8/5. Now, if one sound vibrates eight times in the time it takes another to vibrate five, we get the interval of a minor sixth, which sounds like this:
This is a less basic interval than the perfect fifth, but it’s still part of the fundamental material of music. Like the perfect fifth, it’s a consonant interval, meaning its sounds seem to belong together; the relationship sounds harmonious to our ears. Let’s pause here for a moment to consider what we’re talking about: that some planets orbiting a star unfathomably far away from us are doing so in a way that sounds good to us. Isn’t that something?
What’s more, we’re capable of hearing relationships like 3/2 and 8/5 in other ways than through harmony. As I’ve written about before, pitch and rhythm are the same thing, expressed at different time scales. We can hear 8/5 as an interval between two pitches, and we can also hear it as a rhythm in which one sound is played eight times in the time the other is played five:
What does this rhythm sound like?
Pretty catchy, I would say! What’s important to appreciate is that this is exactly the same as hearing a minor sixth as harmony, only about 200 times slower. Pitch and rhythm are simply two different ways for us to perceive frequency relationships. It’s even possible to experience one turning into the other, and back again:
With the TRAPPIST-1 system, it’s not only the inner and outer two planets that orbit in a resonant harmonic relationships. All seven, surprisingly, do — the only planetary system discovered so far with such consistently resonant orbits. Their relationships, from inner to outer, are very nearly as follows: 8/5, 5/3, 3/2, 3/2, 4/3, and 3/2. This works out to a combined 2 / 3 / 4 / 6 / 9 / 15 / 24. Let’s hear all of these together, built up from the outermost planet to the innermost, in music:
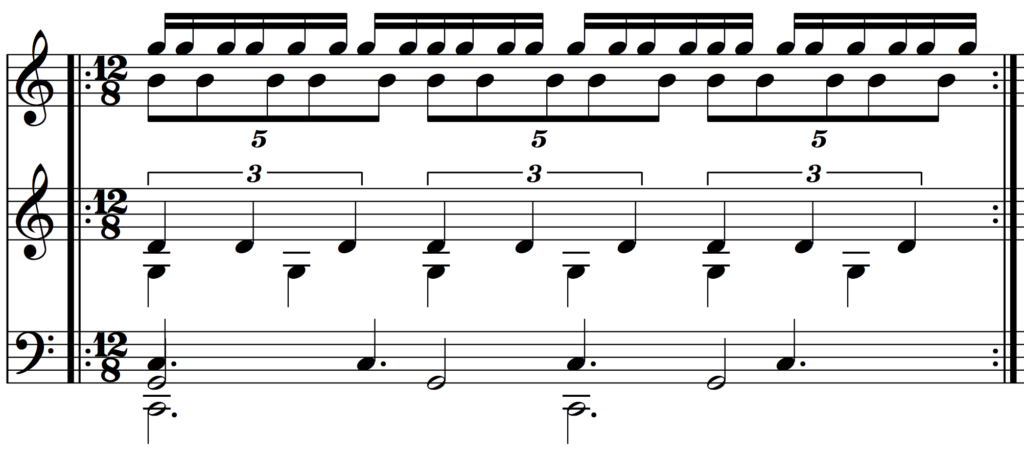
Pretty, right? Now let’s hear these same relationships as rhythm, but instead of playing each note with a percussive sound as we did before with the minor sixth, let’s express each with the pitch that results from the orbital relationships. For fun, here it is in musical notation:
And in sound:
We’re now hearing the TRAPPIST-1 system as pitch and rhythmic relationships, simultaneously. We’re experiencing the same thing in two different ways at once. As a friend of mine pointed out, it’s a bit like tasting roasted cauliflower next to a cauliflower gratin on a bed of cauliflower couscous.
But wait, something’s off — if you’re listening closely, you may have noticed that this is quite a bit more in tune and in time than the sounds and rhythms played in the video at top. This is because, although the TRAPPIST-1 system has relationships that are very close to whole numbers, they’re actually a little off. Nature is funny that way. The outermost planet doesn’t orbit in exactly 18 days but rather 18.766. The second farthest planet goes around in 12.353 days rather than 12. So their relationship isn’t quite 1.5, but rather 1.51918. We’ve been listening to an idealized version of the system so far. Let’s see what the real thing sounds like, with the most precise orbital periods plugged in, first as pitch, then as pitch and rhythm:
It still sounds quite harmonious, if a little sour (it’s worth comparing to the two previous recordings above). The discrepancies are particularly noticeable in the rhythm, which doesn’t line up quite as neatly as before. The intervals making up the harmonies aren’t as clean, either, but they still “work” — we still hear a clear, quite consonant chord (the history of tuning systems tells us that there is always a little lee-way with tuning). One result of recreating this rendering for myself was to notice that there is a wobble, or vibrato, in the piano-like sound that the TRAPPIST-1 physicists used in their video. To my ears, this causes the harmonies to sound a little further out of tune than they actually are. I’m using simpler, more stable sounds in my own rendering.
So — TRAPPIST-1 is remarkable. Without interfering with its orbits in any way, by just presenting the data scaled up to our range of hearing, we hear what we readily identify as harmonious music. But how special is it? To compare, I wanted to listen to our own Solar System. From Mercury’s 88-day orbit to Neptune’s 165-year one, our home planets take a lot longer to orbit the Sun than TRAPPIST-1’s do. But their relationships — Earth to Mars relate by about 17/9, for example — can be rendered in music just as well.
The Solar System is so spread out that it helps to break it up. Let’s begin with the four inner planets, Mercury, Venus, Earth and Mars. Heard on their own, they spell out an eerie kind of Major 7th chord:
Meanwhile the outer planets Jupiter, Saturn, Uranus and Neptune together have something of a half-diminished color:
Now let’s try to hear these together. Because it is so compact, the planetary system of TRAPPIST-1 can fit neatly into the range of frequencies where we comfortably hear harmonies. Not so for the Solar System. TRAPPIST-1’s innermost planet makes only 12 orbits in the time the outermost makes 1, whereas Mercury makes 684 in the time Neptune makes 1, a vastly greater range that stretches our hearing to the limits. If I assign an extremely low note to Neptune, a low 23Hz rumble right at the bottom of our audible range, that puts Mercury’s sound right at the top of what we can hear, a mere dog whistle at 15,736Hz — which younger listeners will have more success hearing than older ones. Even if we’re able to hear this, we just don’t have the perceptual capacity to hear real harmonic relationships between tones spread that far apart.
For that reason, I’ve transposed the inner four planets down by 2 octaves, and the outer four up by 1 octave. As you’ll remember from music theory class, if you were there, this doesn’t alter the resulting harmony (think chord inversions) — it just allows us to hear it. Here is the sound of our Solar System, from Neptune through Mercury:
There’s no doubt when we hear this that the Solar System isn’t anywhere as harmonious as TRAPPIST-1. Not even in the same ballpark. I actually like the sound that results — it has a mystery that I find enticing — but it’s wildly dissonant. While there are some near-resonances in the Solar System — Uranus makes nearly two orbits in the time it takes Neptune to make one, for example — most of them are too far out of tune to qualify as harmonious.
The fact that the TRAPPIST-1 orbits are so obviously harmonious in comparison tells us something important about the physics of the system. The planets are so close together that they would collide with each other if they hadn’t settled into these resonant orbital relationships. Meanwhile, the planets of the Solar System are far enough apart that they face fewer demands on their orbits.
The great astronomer Johannes Kepler, in addition to contributing enormously to the field of astronomy in the 17th century, was also something of an astrologer. He was particularly interested in how a planet’s speed varied during its orbit, which is what he rendered in musical notation in the figure above. He believed that the happiness of life on Earth depended on how harmoniously the orbits of the planets related to one another. He was following in the footsteps of the ancient Greeks, who advocated for a Harmony of the Spheres over 2,000 years ago. It turns out people have been trying to listen to planetary orbits for a very long time; it’s remarkable that we live in a time where we can accurately listen not only to our own, but to planets around other stars as well.
Aristotle and Kepler would have had to believe that life in the TRAPPIST-1 system, if indeed there any, is pretty dandy. It’s hard to imagine a system with more harmonious planets. Or is it? I’ll leave you with a sound rendering I made of the four Galilean Moons of Jupiter, both in pitch and rhythm. Io, Europa, Ganymede and Callisto have orbits that are perhaps even more harmonious than those of TRAPPIST-1, with a beautiful minor key sonority. Three of the moons are in quasi-perfect octaves, a 2 to 1 relationship that is the simplest there is. I guess life must be pretty good there, too.




Hello Dan,
My husband and I attended your presentation at the Astronomy Centre on Thursday evening.I learned a lot,as a non musical person.It was an illuminating evening.As a result,we also attended your concert on Friday evening.Predictably,it was far over my head,but it has aroused my interest in Bach.
That evening,I gave you a note about a book that I am reading.In my rush,I not only gave part of the title wrong,but also misspelled the author’s name.The book is Do Not Say That We Have Nothing.the author is Madeleine Thien.Canadian,2017.Bach and the Goldberg Variations are central to two of the main characters,a composer and a pianist
I hope that you might take a look at it,and. I would love to hear what you think
Dear Lindsay,
Thanks so much for the kind note you left me in Maui, and thanks for the update on the title. I hope to get to it!
My best,
Dan
Thanks for your amazing site. I’ve always wanted to *hear* Kepler’s ideas. Do you know how the Harmonices Mundi musical notation above relates to the ideas below?
http://www.keplersdiscovery.com/Harmonies.html
“Kepler sought harmonic proportions in the Solar System.
Continuing the search for organizing principles first taken up in the Mysterium Cosmographicum, he asked if the greatest and least distances between a planet and the Sun might approximate any of the harmonic ratios, but found they did not.
He looked at the speed of the planets at the points where they move fastest and slowest, noting that movement represents a better analogue to harmonic vibration than distance.
In fact, he found that planets did seem to approximate harmonies with respect to their own orbits. The maximum and minimum speeds of Saturn (measured in terms of arc seconds seen from the Sun) differed by an almost perfect 4/5 ratio (a major third). The extreme motions of Jupiter differed by a 5/6 ratio (a minor third in auditory space). The orbits of Mars, the Earth, and Venus approximated the following harmonies: 2/3 (called a “diapente”) for Mars; 15/16 for Earth, or the difference between mi and fa; and 24/25 for Venus.”